Question

Jackson has a coin collection consisting of quarters and dimes. The total value of his collection is $16.70. His collection consists of eight less quarters than three times the number of dimes. Using the variables q and d to represent the number of quarters in his collection and the number of dimes in his collection respectively, determine a system of equations that describes the situation. Enter the equations below separated by a comma. How many dimes are in his collection? How many quarters are in his collection?

Answer 1

First, remember that:

• a dime values 10 cents;

,

• a quarter values 25 cents.

So, using q for the number of quarters and d for the number of dimes, we have:

`q=3d-8`

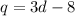
The above equation tells us that the number of quarters is 8 less than three times the number of dimes.

Also, we have:

`0.10d+0.25q=16.70`

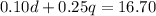
The above equation tells us that the total value of his collection is $16.70.

Thus, the equations are:

`\begin{gathered} q=3d-8 \\ \\ 0.10d+0.25q=16.70 \end{gathered}`

Now, solving that system, we obtain:

`\begin{gathered} 0.10d+0.25(3d-8)=16.70 \\ \\ 0.10d+0.75d-2=16.70 \\ \\ 0.85d=18.70 \\ \\ d=(18.70)/(0.85) \\ \\ d=22 \end{gathered}`

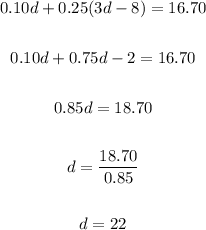
Now, we can use the previous result to find q:

`\begin{gathered} q=3(22)-8 \\ \\ q=66-8 \\ \\ q=58 \end{gathered}`

Therefore, there are 22 dimes and 58 quarters in his collection.