Question

write the first four terms of a decreasing arithmetic sequence. use this to describe, in your own words, how to write the formula for the sequence. then use the formula to calculate the 20th term. show all work

Answer 1

Answer:

The 20th term = -34

Step-by-step explanation:

An arithmetic sequence is one which has a common difference.

For example, the sequence below has a common difference of -2, and first term of 4

4, 2, 0, -2........

The first term, a = 4

The common difference, d = 2 - 4 = -2

The formula for nth term of an arithmetic sequence

`\begin{gathered} a_n=a+(n-1)d \\ \\ a_n=4+(n-1)(-2) \\ \\ a_n=4-2n+2 \\ \\ a_n=6-2n \end{gathered}`

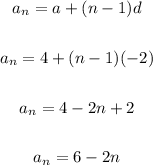
The 20th term is:

`\begin{gathered} a_(20)=6-2(20) \\ \\ a_(20)=6-40 \\ \\ a_(20)=-34 \end{gathered}`

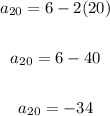
The 20th term = -34