Question

A kangaroo jumps up with an initial velocity of 36 feet persecond from the ground (assume its starting height is 0 feet).Use the vertical motion model, h-16t2 + ut + s, where vis the initial velocity in feet per second and s is the height infeet, to calculate the amount of time the kangaroo is in the airbefore it hits the ground again. Round your answer to thenearest tenth if necessary.Time in air:seconds

Answer 1

Given

Initial velocity:

36 ft/s

Initial height:

0 ft

Vertical motion model:

h(t) = -16t^2 + ut + s

v = initial velocity

s = is the height

Procedure

We are going to use the model provided for the vertical motion.

`\begin{gathered} h(t)=-16t^2+36t+0 \\ h(t)=-16t^2+36t \end{gathered}`

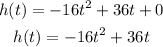
We know that at the maximum height the final velocity is 0.

Then we will use the following expression to calculate the maximum height:

`\begin{gathered} v^2_f=v^2_o-2ah_(\max ) \\ 0=v^2_o-2ah_(\max ) \\ 2ah_(\max )=v^2_o \\ h_(\max )=(v^2_o)/(2a) \\ h_(\max )=((36ft/s)^2)/(2\cdot32ft/s^2) \\ h_(\max )=20.25\text{ ft} \end{gathered}`

Now for time:

`\begin{gathered} 20.25=-16t^2+36t \\ 16t^2-36t+20.25=0 \end{gathered}`

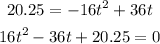
Solving for t,

`\begin{gathered} t_1=2.25 \\ t_2=0 \end{gathered}`

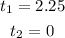
The total time the kangaroo takes in the air is 2.3s.