The line l and line m is parallel to each other and line q is transverse line. So angle 1 and angle 42 are corresponding angles. Thus,
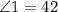
So the corresponding angle postuates is the reason why angle 1 = 42 is True.
The angle 1 and angle 2 has a common arm (or side) and commom vertex. So angle 1 and angle 2 are adjacent angles. The angle 1 angle 2 together form a straight line. So answer is True.
The angle 1 and angle 2 form a straight line. So sum of angles is equal to 180 degrees.
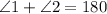
So answer is True.
Substitute 42 for angle 1 in equation to determine the measure of angle 2.

The measure of angle 2 is 138 degrees.