Given:
The cost of mixture per pound, M= $5.91.
The quantity of nuts taken first , n=13 pounds.
The cost of nuts per pound, which was taken first, N=$3.10.
The cost of nuts per pound taken second, P=$7.50.
Let p be the quantity of nuts taken second.
Hence, we can write,
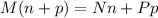
Now, put the values in the above equation.
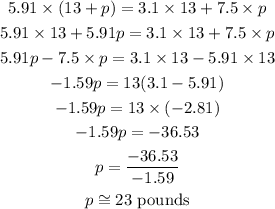
Therefore, 23 pounds of nuts that cost $7.50 per pound is added to make the mixture.