Question

David cast a shadow 42" long at the same place and time in Emma cast a shadow 28" long. David is 66" tall. What is Emma's height?

Answer 1

Emma is 44" tall

Step-by-step explanation

We have that David cast a shadow 42 inches long and he is 66 inches tall.

At that same place and time, Emma cast a shadow of 28 inches.

Since they are at the same spot and time, we can conclude that the ratio of their height to shadow must be the same.

Let Emma's height be x.

The ratio of David's shadow to height is:

42 : 66 or 42 / 66

For Emma, it is:

28 : x or 28 / x

That means that:

`\begin{gathered} (42)/(66)\text{ = }(28)/(x) \\ \text{Solve for x by cross-multiplying:} \\ 42\cdot\text{ x = 66 }\cdot\text{ 28} \\ x\text{ = }\frac{66\cdot\text{ 28}}{42} \\ x\text{ = 44 inches} \end{gathered}`

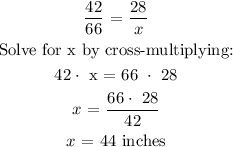
So, Emma is 44" tall.