Question

Using conservation of energy, how fast would a rollercoaster be traveling at a point half way above the ground where it started from, assuming that is started at rest and 95.7 m above the ground?

Answer 1

Given,

The initial height of the rollercoaster, h₁=95.7 m

The height at which the speed of the rollercoaster is required, h₂=h₁/2=47.85 m

From the law of conservation of energy, the total energy of a system will always remain constant. That is, the potential energy lost by the rollercoaster as it descends will be equal to the kinetic energy gained by it.

Thus the kinetic energy of the rollercoaster halfway above the ground is given by,

`\begin{gathered} (1)/(2)mv^2=mg\Delta h \\ =mg(h_1-h_2) \\ =mg(h_1-(h_1)/(2)) \\ =mg(h_1)/(2)_{} \end{gathered}`

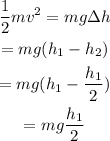
Where m is the mass of the rollercoaster, v is the speed of the rollercoaster halfway above the ground, and g is the acceleration due to gravity.

On further simplifying the above equation,

`\begin{gathered} (1)/(2)v^2=g(h_1)/(2) \\ \Rightarrow v=\sqrt[]{gh_1} \end{gathered}`

On substituting the known values,

`\begin{gathered} v=\sqrt[]{9.8*95.7} \\ =30.62\text{ m/s} \end{gathered}`

Thus the speed of the rollercoaster halfway above the ground from where it started is 30.62 m/s