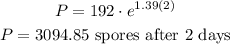Answer:
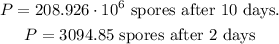
Explanation:
We can models this situation by using exponential growth, which is represented by the following formula:

Therefore, for a starting population of 192 mold spores, and since it quadrupled every day.
After 10 days:
If they quadruple every day, then P/P0=4 when t=1.

Now, after 2 days: