Question

Can you show what number 5 would look like on a unit circle

Answer 1

Given: sin 510⁰

To Determine: The value of the given sines in the unit circle

Solution

To find the value of sin 510 degrees using the unit circle, we will represent 510° in the form (1 × 360°) + 150° [∵ 510°>360°]

Therefore, sine is a periodic function, sin 510° = sin 150°

The value of sin 510 degrees can be calculated by constructing an angle of 510° with the x-axis, and then finding the coordinates of the corresponding point which is (-0.866, 0.5) on the unit circle.

The value of sin 510° is equal to the y-coordinate (0.5).

This is as shown in the image below:

Using radical form

`\begin{gathered} sin(510^0) \\ sin(510^0-360^0)=sin150^0 \\ 150^0lies\text{ in the second quadrant, and sine is positive in the 2nd quadrant} \\ Therefore, \\ sin(510^0)=sin(150^0)=+sin(180^0-150^0) \\ sin(510^0)=sin(150^0)=+sin(30^0) \\ sin(510^0)=sin(150^0)=(1)/(2) \end{gathered}`

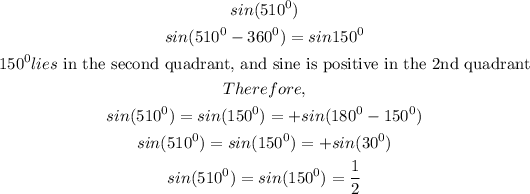
Hence, sin 510° = 0.5 or 1/2