The rule of the average rate of a function f(x) on the interval [a, b] is
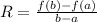
Since the given function is

Then put a = 2 and b = 9, then find f(2) and f(9), and substitute them in the rule above.


Substitute the values of a, b, f(a), and f(b) in the rule above
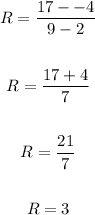
The average rate of change on the given interval is 3