Question

What is the common ratio of the geometric sequence below?-2, 6, -18, 54,

Answer 1

-3

Step-by-step explanation

The formula for the general term of a geometric sequence is:

`a_n=a_1\cdot r^(n-1)`

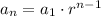
In this sequence the first term a1 = -2. With this and the second term a2 = 6 we can find the common ratio and then verify with the next terms:

`\begin{gathered} a_2=a_1\cdot r^(2-1) \\ a_2=a_1\cdot r \\ r=(a_2)/(a_1) \\ r=(6)/(-2) \\ r=-3 \end{gathered}`

If we use this common ratio to find the 3rd and 4th terms we have to arrive to the same result as the given sequence:

`\begin{gathered} a_3=-2\cdot(-3)^2 \\ a_3=-2\cdot9 \\ a_3=-18\to OK \end{gathered}`
`\begin{gathered} a_4=-2\cdot(-3)^3 \\ a_4=-2\cdot(-27) \\ a_4=54\to OK \end{gathered}`

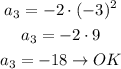
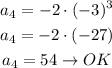
The common ratio of this sequence is -3