Question

Find sum of 91 + 85 + 79 + ... + -29

Answer 1

We have the progression

91, 85, 79, ..., -29.

Also we can test:

85 - 91 = -6

79 - 85 = - 6

Hence, the progression is an arithmetic progression with d = -6.

Now, using the general formula of an arithmetic progression, we can find the position of term -29; as follows:

`\begin{gathered} a_n=a_1+(n-1)* d_{} \\ -29\text{ = 91 + (n - 1)(-6) } \end{gathered}`

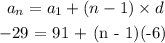
So n = 21

Now we know -29 is the term on the 21 position, we can proceed to find the sum of the first 21st terms of the progression:

`S_n\text{ = }(a_1+a_n)/(2)n\text{ }`

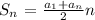
Solving the formula we have:

`S_(21)\text{ = }\frac{91-29_{}}{2}\text{ x 21 }`

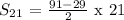
S₂₁ = 651