Answer:
Step-by-step explanation:
a) Firstly, we want to write the growth formula
We have the general form as:
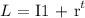
where:
L is the estimated board feet of lumber
l is the is the initial estimated board feet of lumber (the initial estimated board feet of lumber in 2022)
r is the percentage rate of increase which is 9% (9/100 = 0.09)
t is the number of years to reach the estimated board feet of lumber
With respect to the question given, we have the formula as:
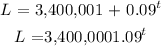
b) We want to get the value of t when L is 1 billion
Substituting the values, we have it that:
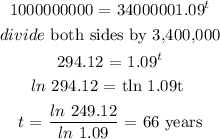
In 66 years (2088) , the groove will reach one billion board feet of lumber