Question

Find the area of a triangle with legs that are: 15 mm, 10 mm, and 20 mm.A. 20.5 mm²B. 16.4 mm²C. 102.7 mm²D. 72.6 mm²

Answer 1

D. 72.6 mm²

Step-by-step explanation:

The area of the triangle can be calculated by Heron's Formula:

`A=\sqrt[]{s(s-a)(s-b)(s-c)}`

Where a, b, and c, are the legs and s can be calculated as:

`s=(a+b+c)/(2)`

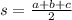
Therefore, replacing a, b, and c by 15, 10, and 20, we get that s is equal to:

`\begin{gathered} s=(15+10+20)/(2) \\ s=(45)/(2) \\ s=22.5 \end{gathered}`

Then, the area of the triangle will be equal to:

`\begin{gathered} A=\sqrt[]{22.5(22.5-15)(22.5-10)(22.5-20)} \\ A=\sqrt[]{22.5(7.5)(12.5)(2.5)} \\ A=\sqrt[]{5273.44} \\ A=72.6mm^2 \end{gathered}`

So, the answer is:

D. 72.6 mm²