To calculate the size of the sample we use the following formula:
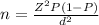
N is the size of the sample, P is the expected proportion of people liking lettuce (36% for this case) and d is the precision, 4% in this case. Z is the Z score for confidence. In this case, 90% of confidence will have a Z value of 1.645.
Then, applying the formula:
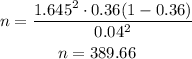
Rounding to the unit, the sample size should be 390.