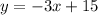Question

Write the equation of a line that is perpendicular to the given line and that passes through the given point. y+2 = 1/3 (x-5); (4,3) y=-3x + 15y=-3x – 9y=-4x+ 3y=-1/3x-11

Answer 1

The equation of a line perpendicular to the given line and passes through the given point is:

`y=-3x+15`

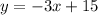
Step-by-step explanation:

Given the line:

`y+2=(1)/(3)(x-5)_{}`

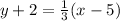
This can be rewritten as:

`\begin{gathered} y=(1)/(3)x-5-2 \\ \\ y=(1)/(3)x-7 \end{gathered}`

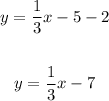
This is a line with slope 1/3, and y-intercept -7.

A line perpendicular to this line has it's slope as the negative reciprocal of 1/3.

The negativen reciprocal of 1/3 is -3.

So, the line is of the form:

`y=-3x+b`

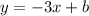
We can find the y-intercept b, by using the given point, (4, 3) in the perpendicular equation we formend.

Where x = 4, and y = 3

Doing this, we have:

`\begin{gathered} 3=-3(4)+b \\ 3=-12+b \end{gathered}`

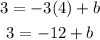
Add 12 to both sides

`b=3+12=15`

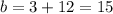
Therefore, the equation of a line perpendicular to the given line and passes through the given point is:

`y=-3x+15`