Question

there's 2 parts of the question.Also , one of the last tutors did multiple questions and helped me with about most of my homework... can you help me with other questions than this too , thank you anyway though.

Answer 1

Part A:

In 2000, the population was 6.26 million.

In 2015, the population was 7 million.

The general form of the exponential function is given by

`A=A_0e^(k\cdot t)`

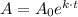
Where A0 is the initial population (6.26 million), k is the growth rate, and t is the number of years after 2000.

Let us first find the growth rate.

We are given that In 2015, the population was 7 million.

`\begin{gathered} A=A_0e^(k\cdot t) \\ 7=6.26e^(k\cdot15) \\ (7)/(6.26)=e^(k\cdot15) \\ \ln ((7)/(6.26))=\ln (e^(k\cdot15)) \\ 0.1117=k\cdot15 \\ k=(0.1117)/(15) \\ k=0.0074 \\ k=0.01 \end{gathered}`

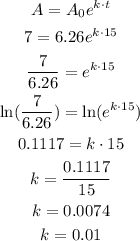
So, the growth rate is 0.01 (rounded to 2 decimal places)

Therefore, the exponential growth model is

`A=6.26e^(0.01t)`

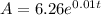
Part B:

We need to find the year for which the population will be 12 million.

Let us substitute A = 12 into the exponential growth function and solve for t

`\begin{gathered} A=6.26e^(0.01t) \\ 12=6.26e^(0.01t) \\ (12)/(6.26)=e^(0.01t) \\ \ln ((12)/(6.26))=\ln (e^(0.01t)) \\ 0.6507=0.01t \\ (0.6507)/(0.01)=t \\ 65=t \\ t=65\: \text{years} \end{gathered}`

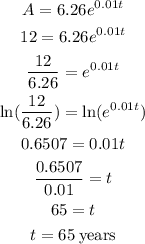
Therefore, the country's population will be 12 million in the year 2065