Question

3. Find the value of x for which ABCD must be a parallelogram(X+3)*(2x - 1)

Answer 1

For the shape ABCD to be a parallelogram, the sides AB and CD must be parallel:

`AB||CD`

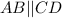
Hence, line BD is the transversal.

Note that angles ABD and CDB are alternate interior angles. By definition, the angles will be equal:

`m\angle ABD=m\angle CDB`

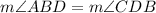
Therefore, substituting the values of the angles will yield the following equation:

`x+3=2x-1`

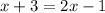
Solving the equation for x:

`\begin{gathered} 2x-x=3+1 \\ x=4 \end{gathered}`

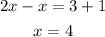
Therefore, the correct option is the THIRD OPTION.