Question

How do i solve and what’s the answer for number 14?

Answer 1

Hello!

We have the following expression:

`\sum_{n\mathop{=}0}^6300(1.05)^(n-1)`

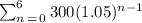
We can solve it as a geometric sequence, I'll show you how.

First, let's write the number of terms of the sequence:

Starting in 0 until 6, we have: a1, a2, a3, a4, a5, a6 and a7.

(7 terms).

Also, we can see that the ratio is 1.05.

Now, let's calculate the first term of the sequence (a1):

`\begin{gathered} a_1=300*(1.05)^(0-1) \\ a_1=300*(1.05)^(-1) \\ a_1=300*(1)/(1.05) \\ a_1=(2000)/(7) \end{gathered}`

As we know the first term of the sequence and the ratio, we can use the formula below to calculate the sum of the 7 terms of this sequence:

`\boxed{S_n=(a_(1)(r^(n)-1))/(r-1)}`

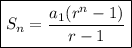
So, let's replace it with the values that we already know:

`S_7=((2000)/(7)(1.05^7-1))/(1.05-1)=((2000)/(7)(1.4071-1))/(0.05)=((2000)/(7)(0.4071))/(0.05)\cong2326.29`

Right answer: alternative B.