The value of the acceleration given is,
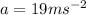
The angle of the acceleration with the negative of the y-axis in the third quadrant is,
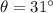
Solution:
The diagrammatic representation of the given system is,
The angle of the acceleration with the positive of the x-axis is,
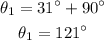
Thus, the component of the acceleration along the positive of the x-axis is,

The angle of the acceleration with the positive of y-axis is,
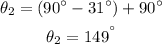
The y-component of the acceleration is,
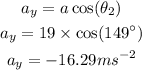
Thus, the x and y-component of the acceleration are -9.8,-16.29.