ANSWERS
• x = 6√3
,
• y = 12
Step-by-step explanation
This is a right triangle. We know the measure of one of the angles, and the length of the leg adjacent to this angle. We have to find the length of the opposite leg and the length of the hypotenuse. To find these, we will use trigonometric ratios,

Multiply both sides by 6 to solve for x,
![x=6\tan 60\text{\degree}=6\cdot\sqrt[]{3}](https://img.qammunity.org/2023/formulas/mathematics/college/2pt45wip0ynd4evfs1iaoz63kmpesrpm6p.png)
For x we will use the cosine,
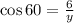
Solve for y,
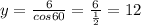
Hence, x = 6√3 and y = 12.