Question

Consider the line 3x + 4y = 71. what is the slope of a line perpendicular to this line?2. what is the slope of a line parallel to this line?

Answer 1

The equation of the line is given as

`3x+4y=7`

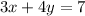
The general equation of a line is

`\begin{gathered} y=mx+c \\ \text{where,} \\ m=\text{slope} \\ c=\text{intercept on the y a}\xi s \end{gathered}`

Step 1: Make y the subject of the formula

`3x+4y=7`

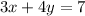
Subtract 3x from both sides

`\begin{gathered} 3x+4y=7 \\ 3x-3x+4y=7-3x \\ 4y=-3x+7 \end{gathered}`

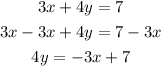
Step 2: Divide all through by 4

`\begin{gathered} 4y=-3x+7 \\ (4y)/(4)=-(3x)/(4)+(7)/(4) \\ y=-(3x)/(4)+(7)/(4) \end{gathered}`

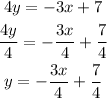
Step 3 : Compare coefficients with the general equation of a line below

`\begin{gathered} y=mx+c \\ y=-(3x)/(4)+(7)/(4) \\ \text{hence,} \\ m=-(3)/(4),c=(7)/(4) \end{gathered}`

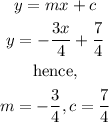
For a perpendicular line,

`m_1* m_2=-1`

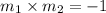
That is, we will have that

`\begin{gathered} -(3)/(4)* m_2=-1 \\ -(3m_2)/(4)=-1 \\ \text{cross multiply.} \\ -3m_2=-4 \\ \text{divide both sides by -3} \\ (-3m_2)/(-3)=(-4)/(-3) \\ m_2=(4)/(3) \end{gathered}`

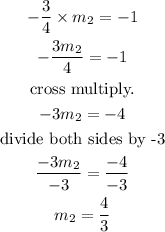
Hence,

The slope of the perpendicular line will be = 4/3

For a parallel line,

`m_1=m_2`

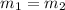
Therefore,

The slope of a parallel line will be = -3/4