a)
Since the weights follow a normal distribution this mean we can use it to find the proportions. In this case the proportion of birth wights above 125 oz is the same as finding the probability:

To determine the probability we need to use the standard normal distribution, defined by the z-score:

where x is the value we are looking for, mu is the mean and sigma is the standard deviation.
Then the probability stated above takes the form:
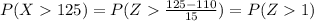
Now, looking at a standard normal distribution table we have:
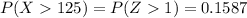
Therefore we conclude that 15.87% of the birth weights is above 125 oz.
b)
In this case we are looking for the probability:
[tex]P(125Using the proabability distribution properties we have that:
[tex]P(125transforming this probabilities to z-scores and looking at a table we have:
[tex]\begin{gathered} P(125
Therefore the proportion of weights between 125 and 140 oz. is 13.59%