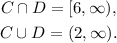First, we know that set C consists of all real numbers greater than 2, in interval notation we have that:
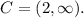
Now, we also know that D consists of all real numbers greater or equal to 6, in interval notation we have that:
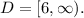
Therefore, for a number to be in the intersection of both sets, the number has to be greater than 2 and greater or equal to 6, this implies that the number must be greater or equal to 6 to fulfill both conditions. On the other hand, if we want to find a number in the union of C and D, it is enough for the number to be greater than 2 or greater or equal to 6, therefore a number in the union must be greater than 2.
Answer: