Question

The GMAT scores of all examinees who took that test this year produced a distribution that is approximately normal with a mean of 460 and a standard deviation of 31.The probability that the score of a randomly selected examinee is more than 530, rounded to three decimal places, is:Type your answer here

Answer 1

Given:

a.) Approximately normal with a mean of 460 and a standard deviation of 31.

b.) The probability that the score of a randomly selected examinee is more than 530.

Step 1: Let's determine the z-score.

`\text{ z-score = }(x-\mu)/(\sigma)`
`\text{ = }\frac{530\text{ - 460}}{31}`
`\text{ = 2.258064516129032}`
`\text{ z-score }\approx\text{ 2.26}`

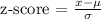
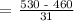
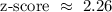
Step 2: Let's use the z-score table to determine its equivalent probability.

From the given chart, it appears that at z-score of 2.26, the probability is 0.9881

Therefore, the answer is 0.9881 or 98.81%