Question

you have 1000 to invest in an account with a rate of 8%, compounded semi annually. how long will it take you to double your money

Answer 1

Given:

Initial Amount, P = $1000

Rate of interest, r = 8% = 0.08

Number of years, t = ?

Number of compounding period, n = 2

Required: Time required to double the initial amount.

Step-by-step explanation:

The formula to find the compound amount is

`A=P(1+(r)/(n))^(nt)`

Since, A is the double of initial amount, A = $2000

Substitute the given values into the formula.

`\begin{gathered} 2000=1000(1+(0.08)/(2))^(2\cdot t) \\ 2=1.04^(2t) \end{gathered}`

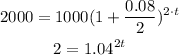
Take logarithm with base 1.04 on both sides.

`\begin{gathered} \log_(1.04)2=\log_(1.04)(1.04^(2t)) \\ 2t=17.67 \\ t=8.835 \end{gathered}`

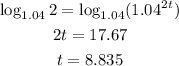
Thus, 8.835 years required to double the initial deposit.

Final Answer: 8.835 years required to double the initial deposit.