The graph of this distribution is:
Now, from it we know that the probability has to be less 0.1586 but more than 0.0227.
To find the probability we have to use the z-score to transform our distribution to a standard normal distribution.
The z-score is given by:

Then in this case we have:
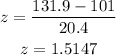
Then our probability is:
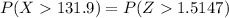
Looking on a table for the value of the probability we have that:

Therefore the probability is 0.0649; which is the same as 6.49%.