Answer:
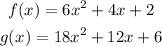
Step-by-step explanation:
Definition:
The degree of a polynomial is the highest index/power of the variable in the polynomial.
The quotient of two polynomials will have a degree of zero if they are multiples of one another.
Let the two polynomial functions be:
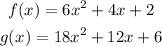
Its quotient:
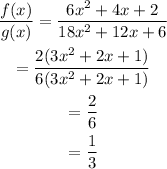
Note:
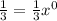
Since the quotient is a constant, it has a degree of zero.