Answer:
• x-intercept = 10
,
• y-intercept = 10/3
Step-by-step explanation:
Given the points:

First, determine the equation of the line using the two-point form:
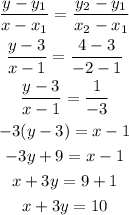
Next, express it in the intercept form:
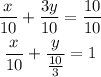
Therefore, the intercepts are:
• x-intercept = 10
,
• y-intercept = 10/3
,
•