Question

11A study of college football games shows that the number of holding penalties assessed has a mean of 2.3 penalties per game and a standard deviation of 1.1 penalties per game.What is the probability that, for a sample of 40 college games to be played next week, the mean number of holding penalties will be 2.15 penalties per game or less?Carry your intermediate computations to at least four decimal places. Round your answer to at least three decimal places.

Answer 1

We are going to use the normal standard distribution to resolve this exercise, in that sense, finding the z value is necessary. Doing the calculations, we get

`\begin{gathered} z=\frac{\frac{x-\bar{x}}{s}}{√(n)} \\ z=((2.15-2.3)/(1.1))/(√(40)) \\ z=((-3)/(22))/(2√(10))\approx-0.0215609 \end{gathered}`

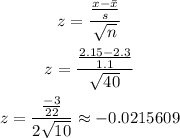
Now it only remains to calculate the probability, using a normal distribution calculator or a normal distribution table as follows

`P(z<-0.0216)\approx0.4914`

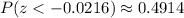
Thus, the probability that, for a sample of 40 college games to be played next week, the mean number of holding penalties will be 2.15 penalties per game or less is 0.4914