In order to calculate the interest rate compounded continuously, we can use the formula:
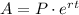
Where A is the final amount after t years, P is the principal and r is the interest rate.
So, using A = 2P, P = 12500 and r = 0.09, we have:
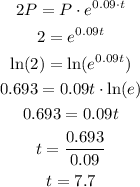
It will take 7.7 years to double the initial investment.