Question

IQ is normally distributed with a mean of 100 and a standard deviation of 15. Supposed one individual is randomly chosen. Let X = IQ of an individual.

Answer 1

Given:

mean(μ) = 100

SD (σ) = 15.

Find: the IQ interval that covers the middle 80%

Solution:

The middle 80% would cover 40% to the right and 40% to the left of the mean.

Using the standard normal distribution table, locate the z-value that covers 40% from the center.

Based on the table, the z-value that covers 40% from the center is +1.282 to the right and -1.282 on its left.

Now, to get the exact IQ values located on these z-values, let's use the formula below.

`x=z\sigma+\mu`

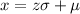
Let's plug into the formula above, mean(μ) = 100 and SD (σ) = 15.

At z = 1.282,

`\begin{gathered} x=(1.282)(15)+100 \\ x=19.23+100 \\ x=119.23\approx119 \end{gathered}`

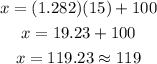
At z = -1.282,

`\begin{gathered} x=(-1.282)(15)+100 \\ x=-19.23+100 \\ x=80.77\approx81 \end{gathered}`

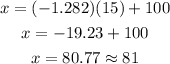
Therefore, the middle 80% of IQs fall between 81 and 119.

x₁ = 81

x₂ = 119