Question

For f(x) = 1/x-5 and g(x)= x² +2, find:Part 1:A. Find the expression for g(x). B. Substitute the value of g(x) into the function f(x) in place of x to find thevalue of f(g(x)). Part II: (gof)(6)A. Find f(6). B. Substitute the value you found in Part I into g(x) to find g(f(6)).

Answer 1

The Solution:

Given:

`\begin{gathered} f(x)=(1)/(x-5) \\ \\ g(x)=x^2+2 \end{gathered}`

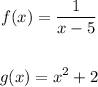
Required:

Part II:

Find the values of:

`gof(6)`

Step 1:

Find g(f(x) by substituting f(x) in the place x in g(x).

`g(f(x))=((1)/(x-5))^2+2`
`g(f(x))=(1)/((x-5)^2)+2`

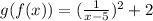
Step 2:

Find the value of g(f(6)).

Substitute x = 6 in g(f(x)).

`g(f(6))=(1)/((6-5)^2)+2=(1)/(1^2)+2=1+2=3`

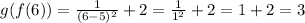
Alternatively:

Substitute x = 6 in f(x).

`f(6)=(1)/(6-5)=(1)/(1)=1`

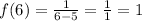
Substitute f(6) = 1 in g(x) to get g(f(6)).

`g(f(6))=g(1)=1^2+2=1+2=3`

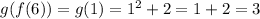
Answer:

g(f(6)) = 3