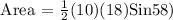Question

Which expressions correctly represent the area of triangle XYZ. XY=14, YZ=10, ZX=18, angle X=38 degrees angle Z=58 degrees and h is unknown.

Answer 1

Let's put more details in the given figure to better understand the problem:

The scenario is that we are tasked to find the area of the triangle with the height not given.

Recall: The formula for getting the area of the triangle.

`\text{ Area = }(1)/(2)(\text{Base\rparen\lparen Height\rparen}`

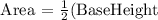
Getting the area, it involves the height of the triangle. Thus, since the other sides were given, we can get the height using the principle of trigonometric functions.

We can get the height in two ways, at Triangle A and Triangle B.

For triangle A:

`\text{ Sine \lparen38}\degree)\text{ = }\frac{\text{h}}{14}`
`\text{ h = 14Sin\lparen38}\degree)`

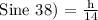
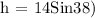
For triangle B:

`\text{ Sin\lparen58}\degree)\text{ = }\frac{\text{h}}{\text{10}}`
`\text{ h = 10Sin\lparen58}\degree)`

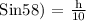
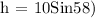
Thus, we can also get the area of the triangle in two ways,

Area 1 (Triangle A perspective):

`\text{ Area = }(1)/(2)bh\text{ = }(1)/(2)(14)(18)\text{Sin\lparen38}\degree)`

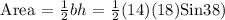
Area 2 (Triangle B perspective):

`\text{ Area = }(1)/(2)(10)(18)\text{Sin\lparen58}\degree)`