Question

What is the perimeter of a rectangle with vertices at (3,2) (-2,2) (-2,-2) and (3,-2

Answer 1

To find the perimeter

Let ABCD be the vertices of (3,2) (-2,2) (-2,-2) and (3,-2​) respectively

A(3,2) B (-2,2) C(-2,-2) and D (3,-2)

​

We will find the distance AB, BC, CD and DA

Using the distance formula;

`|d|=\sqrt[]{(x_2-x_1)^2+(y_2-y_1)^2}`

Find the distance AB

A(3,2) B (-2,2)

x₁ = 3 y₁=2 x₂=-2 y₂=2

substituting the value into the formula:

`|AB|=\sqrt[]{(-2-3)^2+(2-2)^2}`

Evaluate

`|AB|=\sqrt[]{(-5)^2+0}`
`=\sqrt[]{25}`

`=5`

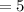
Find the distance BC

B (-2,2) C(-2,-2)

x₁ = -2 y₁=2 x₂=-2 y₂=-2

Substitute into the formula

`|BC|=\sqrt[]{(-2+2)^2+(-2-2)^2}`

Evaluate

`=\sqrt[]{0+(-4)^2}`

`=\sqrt[]{16}`
`=4`

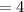
Find the distance CD

C(-2,-2) D (3,-2)

x₁ = -2 y₁=-2 x₂=-3 y₂=-2

Substitute into the formula

`|CD|=\sqrt[]{(-3+2)^2+(-2+2)^2}`

Evaluate

`|CD|=\sqrt[]{(-5)^2+0}`
`=\sqrt[]{25}`
`=5`

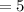
Find the distance DA

D(3 -2) A(3, 2)

x₁ = 3 y₁=-2 x₂=3 y₂=2

Substitute into the formula

`|DA|=\sqrt[]{(3-3)^2+(2+2)^2}`

Evaluate

`=\sqrt[]{0+4^2}`
`=\sqrt[]{16}`
`=4`

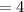
To get the perimeter, we will add all the distance

That is:

Perimeter = AB + BC + CD + DA

=5 + 4 + 5 + 4

=18

Perimeter = 18