Question

Complete combustion of 6.30 g of a hydrocarbon produced 20.5 g of CO2 and 6.29 g of H2O. What is the empirical formula for the hydrocarbon? Insert subscripts as necessary.

Answer 1

CH₂

Step-by-step explanation

It is important to note that you are dealing with a hydrocarbon, that is, a compound that contains only carbon and hydrogen.

Since the products of this combustion reaction are carbon dioxide, CO2, and water, H2O, this let you know that all the carbon that was initially a part of the hydrocarbon will now be part of the carbon dioxide. Likewise, all the hydrogen that was initially a part of the hydrocarbon is now a part of the water.

To determine how many moles of carbon and of hydrogen were originally present in the hdyrocarbon, this means that you can use the number of moles of water and carbon dioxide, respectively, as follows.

For water

`6.29g*\frac{1\text{ }mole\text{ }H_2O}{18.015g}=0.34915\text{ moles }H_2O`

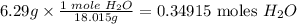
For CO2

`20.5g*\frac{1\text{ mole }C_{}O_2}{44.01g}=0.46580\text{ moles of CO}_2`

Every mole of water contains two moles of H and one mole of O. This implies which means that the reaction produced

`0.34915\text{ moles }H_2O*\frac{2\text{ moles H}}{1\text{ mole }H_2O}=0.69830\text{ moles of H}`

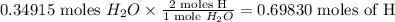
Every mole of carbon dioxide contains 1 mole of carbon and 2 moles of oxygen, it follows that the reaction also produced

`0.46580\text{ }moles\text{ }CO_2*\frac{1\text{ mole of C}}{1\text{ mol}e\text{ of }CO_2}=0.46580\text{ moles of C}`

Lastly, to find the mole ratio that exists between carbon and hydrogen in the hydrocarbon, divide the mole values by the smallest one.

`\begin{gathered} \text{For C: }\frac{0.46580\text{ moles}}{0.46580\text{ moles}}=1 \\ \\ \text{For H: }=\frac{0.69830\text{ mol}es}{0.34915\text{ moles}}=2 \end{gathered}`

The empirical formula of the hydrocarbon will be C₁H₂ ⇒ CH₂

.