ANSWER
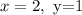
Step-by-step explanation
Let the hot dog be represented by x,
Let (y) represent soda
For "two hot dogs and three soda costs $7" this means;

For "the cost of four hot dogs and two sodas is $10" we have;
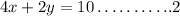
Now, using elimination method, we multiply equation 1 by 2 and equation 2 by 1 so as to eliminate x
Hence, we have;
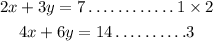
Now to eliminate x, we subtract equation 2 from 3
so, we have;
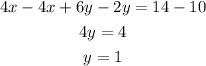
Now substitute the value of y into equation 1 to get x
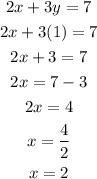
Therefore the cost of 1 hot dog is $2 while that of soda is $1