In this case, we'll have to carry out several steps to find the solution.
Step 01:
data:
point 01 (-3 , 4)
point 02 (5 , 2)
Step 02:
equation of the line:
slope:
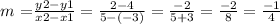
Point-slope form of the line:
(y - y1) = m (x - x1)
(y - 4) = -1 / 4 (x - (-3))
(y - 4) = -1 /4 (x + 3)
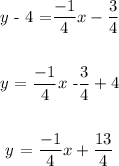
The answer is:
