Solution
- The triangles ABD and BDC share a common side, BD and have sides AB and BC equal.
- Since two sides from both are equal, then, we can conclude that their third sides are equal as well.
- Thus, we can equate the expression for the sides AD and DC
- We have:
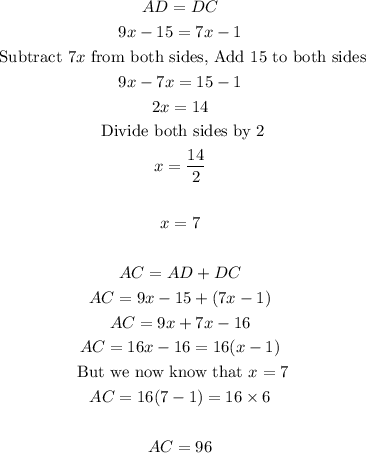
Final Answer
x = 7
AC = 96