Given
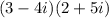
To solve the operation you have to distribute the multiplication, which means that you have to multiply each term of the first parentheses with each term of the second parentheses

Now we know that the imaginary number "i" is equal to the square root of -1, so the square of i is equal to -1:
![\begin{gathered} i=\sqrt[]{-1} \\ i^2=(\sqrt[]{-1})^2 \\ i^2=-1 \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/7e34xb7s2ojicg4urie766evrckq4j1av1.png)
Knowing this, we can simplify the result further:
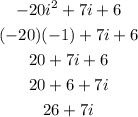
The result of the operation is 26 + 7i