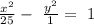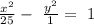
Step-by-step explanation:
Equation of hyperbola:

The vertices are (-5, 0) and (5, 0)
a = 5
-a = -5
Hence, a = 5
a² = 5² = 25
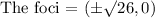
foci = (26, 0) and (-26, 0)
c = √26
-c = -√26
So, c = √26
c² = (√26)² = 26
To get b:
c² = a² + b²
b² = c² - a²
b² = 26 - 25
b² = 1
b = √1 = +/- 1
Inserting the values the equation above: