Solution:
Given the system of equations:
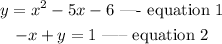
To find the solutions to the system of equations,
step 1: From equation 2, make y the subject of the formula.
Thus,
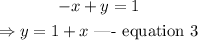
step 2: substitute equation 3 into equation 1.
Thus,
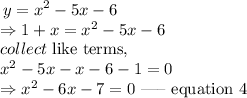
step 3: Solve for x in equation 4.
By factorization,
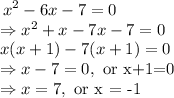
Step 4: Substitute the values of x into equation 3.
Thus, from equation 3
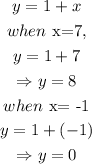
Hence, the solutions to the system of equations are
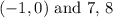
The second option is the correct answer.