We have an equation h(t) describing the height of the projectile:
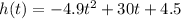
We have to find the time t when it hits the ground.
Mathematically, this means:
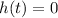
Then, t will be one the roots of the quadratic equation h(t) (NOTE: the other root will be an invalid value of t).
We can calculate the root as:
![\begin{gathered} t=\frac{-30\pm\sqrt[]{30^2-4\cdot(-4.9)\cdot4.5}}{2.\cdot(-4.9)}_{} \\ t=\frac{-30\pm\sqrt[]{900+88.2}}{-9.8} \\ t=\frac{30\pm\sqrt[]{988.2}}{9.8} \\ t\approx(30\pm31.44)/(9.8) \\ t_1\approx(30-31.44)/(9.8)=(-1.44)/(9.8)=-0.15 \\ t_2\approx(30+31.44)/(9.8)=(61.44)/(9.8)=6.27 \end{gathered}](https://img.qammunity.org/2023/formulas/mathematics/college/7duieqlrisxyfbwvgcl6uce0gcsv2vjwo0.png)
The root t = -0.15 is not valid, as t has to be greater than 0.
Then, the projectile will hit the ground after t = 6.3 seconds.
Answer: 6.3 seconds.