If y varies directly as x, then they have a proportional relationship that can be written as:

where k is a constant.
We know that, when x = 8, y = 120.
We can find the value of k, but as we only need to find the value of y when x = 13, we wil use a property of proportional relationships:
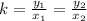
This property tells us that the ratio y/x is constant for all pairs (x,y). Then, we can write:
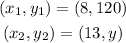
Then, we can write the ratios as:
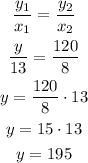
NOTE: that we inplicitly calculated the value of k, that is k = 15. Then we know that the relation is y = 15x.
Answer: y = 195 when x = 13.