Question

Graph each function using RADIANS. State the amplitude, period and midline

Answer 1

For the function:

`y=-2+\sin \theta`

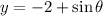
The graph of the function is as shown below:

The Amplitude is the maximum displacement of the graph function from the rest position.

Thus, the amplitude is evaluated as

`\begin{gathered} Amplitude=-1-(-2_{}) \\ =-1+2 \\ Amplitude=1 \end{gathered}`

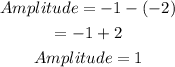
The period of the function is the distance between any repetition of the function.

Thus,

`\begin{gathered} \text{Period = 2}\pi-\pi \\ \Rightarrow Period=\pi \end{gathered}`

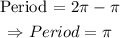
The midline of the function graph is the horizontal line about which the graph function oscillates.

Thus, the midline of the graph is

`y=-2`