Question

8. Suppose that the time that a new roof will last before needing to be replaced follows a normal distribution, with a mean of 25 years and a standard deviation of 5 years. Estimate the percentage of roofs that will last(a) between 15 and 35 years. %(b) longer than 35 years. %(c) less than 20 years. %(d) between 10 and 35 years. %

Answer 1

Problem Statement

The question tells us that the number of years new roofs last follows a normal distribution with a mean of 25 and a standard deviation of 5 years.

We are asked to find the following percentages of:

a) between 15 and 35 years.

b) longer than 35 years.

c) less than 20 years

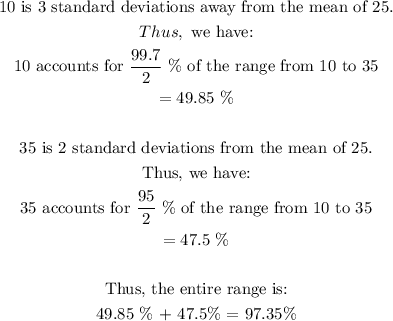
d) between 10 and 35 years.

Method

To solve this question, we need to take note of two formulas. The first is how to calculate the z-score of the individual years, and the other is how to calculate the z-score for a range of years.

Z-score for Individual years:

`\begin{gathered} Z=(X-\mu)/(\sigma) \\ \text{where,} \\ X=\text{individual year} \\ \mu=\text{mean of the normal distribution} \\ \sigma=\text{standard deviation} \end{gathered}`

Z-score for a range of years:

c) less than 20 years:

`\begin{gathered} 20\text{ years is 1 standard deviation from the mean.} \\ \text{Thus, we have:} \\ (100-68)/(2)*100=16\text{ \%} \end{gathered}`

d) between 10 and 35 years:

`\begin{gathered} 10\text{ is 3 standard deviations away from the mean of 25.} \\ Thus,\text{ we have:} \\ 10\text{ accounts for }(99.7)/(2)\text{ \% of the range from 10 to 35} \\ =49.85\text{ \%} \\ \\ 35\text{ is 2 standard deviations from the mean of 25.} \\ \text{Thus, we have:} \\ 35\text{ accounts for }(95)/(2)\text{ \% of the range from 10 to 35} \\ =47.5\text{ \%} \\ \\ \text{Thus, the entire range is:} \\ 49.85\text{ \% + 47.5\% = 97.35\%} \end{gathered}`