SOLUTION
Circumference is the distance around a plane shape. So, it also measures length.
Length and area of two similar shapes are related by the formula

Where the A in both cases represents area and the L represents length. But here the Ls will represent circumference.
Since the circumference of the circle increased by 50%, then the new circumference becomes
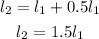
So, we have
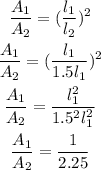
This becomes
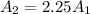
Now subtracting from the original area then
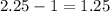
This means that the area was increased by 1.25, which means 125%
Hence option B is the correct answer