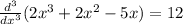Answer:
The indicated derivative of the function would be 12
Step-by-step explanation:
According to the given data we have the following function:
y=2x^3 + 2x^2 - 5x
To calculate third derivative of the function we would have to make the following calculations:
First find the first derivative of y=2x^3 + 2x^2 - 5x
So:
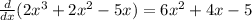
Next, we would have to find the derivative of 6x^2 +4x -5
So:
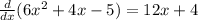
Finally we would have to find the derivative of 12x+4
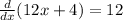
Therefore: