Identify the angle of incidence and the angle of refraction when the ray passes from the transparent substance to the air:
The refractive index of the transparent substance is 1.09 and the refractive index of air is close to 1. Then:
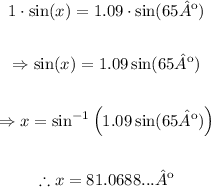
The angle α is complementary to the angle x. Then:
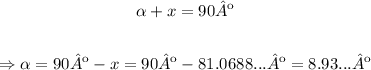
Therefore, the angle α is equal to 8.93º.