The midpoint is given by the following expression:
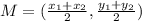
Where:
Therefore:
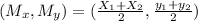
Replacing:
Mx= 5 and My=6
Qx= X1 =-1 and Qy= y1=3
Rx=X2 and Ry= Y2

Equating the x component and solving for X2:
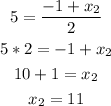
Doing the same with the y component:

Answer: the coordinates of R are: (X2,Y2) = (11 , 9).